If not i would appreciate if someone could show me why the answer i am getting is wrong and if so how to get the corr.
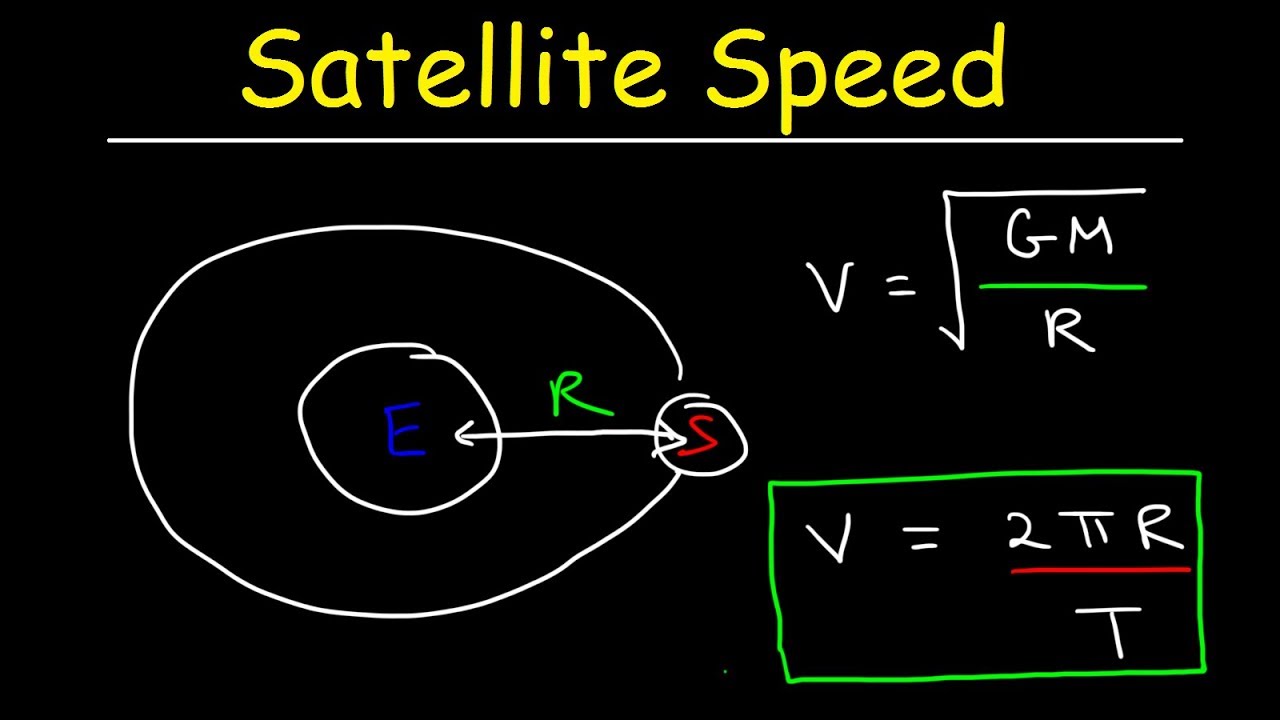
Linear velocity of a satellite in a circular orbit is.
Listed below is a circular orbit in astrodynamics or celestial mechanics under standard assumptions.
Here the centripetal force is the gravitational force and the axis mentioned above is the line through the center of the central mass perpendicular to the plane of motion.
What is the orbital radius.
The orbital velocity of the international space station is 7672 m s.
Below the characteristics of a small satellite orbiting a massive planet at uniform speed in perfectly circular orbit are derived.
A satellite traveling in a circular orbit 8000km from the earth s center takes 2 hours to make an orbit.
Planet moon artificial satellite spacecraft or star is the speed at which it orbits around either the barycenter or if one object is much more massive than the other bodies in the system its speed relative to the center of mass of the most massive body.
Now this r is the sum of the radius of the earth r and the height h of the satellite from the surface of the earth.
The orbital radius can be found by rearranging the orbital velocity formula.
However when a small object like a satellite asteroid or small moon orbits a large object like a planet or star it is a good approximation to treat the system as a two body system with the larger body fixed.
Here m is the mass of earth and m is the mass of the satellite which is having a uniform circular motion in a circular track of radius r around the earth.
2 a satellite is orbiting the earth with an orbital velocity of 3200 m s.
Taking the square root of each side leaves the following equation for the velocity of a satellite moving about a central body in circular motion.
In gravitationally bound systems the orbital speed of an astronomical body or object e g.
Where g is 6 673 x 10 11 n m 2 kg 2 m central is the mass of the central body about which the satellite orbits and r is the radius of orbit for the satellite.
R 3 897 x 10 7 m the orbital radius for this satellite is 3 897 x 10 7 m.
This physics video tutorial explains how to calculate the speed of a satellite in circular orbit and how to calculate its period around the earth as well.
V is the linear velocity of the satellite at a point on its circular track.
I have a problem from a sample final i believe they have incorrect answers.